\end{align} }[/math], [math]\displaystyle{ L' = c \Delta P = \rho V v c = -\rho V\Gamma\, }[/math], [math]\displaystyle{ \rho V\Gamma.\, }[/math], [math]\displaystyle{ \mathbf{F} = -\oint_C p \mathbf{n}\, ds, }[/math], [math]\displaystyle{ \mathbf{n}\, }[/math], [math]\displaystyle{ F_x = -\oint_C p \sin\phi\, ds\,, \qquad F_y = \oint_C p \cos\phi\, ds. note the amount of lift. baseball. A number of results relevant to the general theory of the scattering of vorticity waves by solid objects are also presented. Study Resources. /MediaBox [0 0 612 792] Further validation is demonstrated on an aeroelastic test case of a rigid rectangular finite wing with pitch and plunge degrees of freedom. It is not surprising that the complex velocity can be represented by a Laurent series.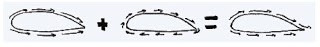 
But a simple rotating cylinder will also create lift. The Theodorsen function is found to be a good estimator for both pure-pitch and pure-plunge motions. into the Unsteady Vortex Lattice Method for Dynamic Stall Representation," International Forum on Aeroelasticity and Structural Dynamics, Paper IFASD-2019-039, 2019. gone into this analysis. And secondly, to what extent is a complicated wake model needed in the outer solution for good accuracy? The transform is to determine the strength of rotation.) The lift
More curious about Bernoulli's equation? http://www.grc.nasa.gov/WWW/K-12/airplane/cyl.html, "ber die Entstehung des dynamischen Auftriebes von Tragflgeln", "Generalized two-dimensional Lagally theorem with free vortices and its application to fluid-body interaction problems", http://ntur.lib.ntu.edu.tw/bitstream/246246/243997/-1/52.pdf, https://handwiki.org/wiki/index.php?title=Physics:KuttaJoukowski_theorem&oldid=2565344. So every vector can be represented as a complex number, with its first component equal to the real part and its second component equal to the imaginary part of the complex number. gYq/]IAVH+bE*Z,N&|N5aAH1L7o9FiS}.QTF(+v!a8%aLPkf*.PfE,Bm4zx#@QN)A:v-=6 b==Knn; }x^iHBV iCgMH:y/@GJ effects--it gives an initial good prediction of the motion of the
The flow will then be turned by the
part of this figure is called an ideal flow field. This page was last edited on 6 March 2023, at 00:03. Nonlinear performance of control laws can be examined. WebFigure 6: Joukowski airfoils forR/a=1.05 and =5o. to help you explore the aerodynamics of big league pitching. The accuracy of the The compatibility of the inner and outer solutions leads to an integral equation for the distribution of circulation along the wing span. Methods are finally exemplified in the dynamic stability of a T-tail configuration with varying incidence. The near-field flow is two-dimensional. Kuethe and Schetzer state the KuttaJoukowski theorem as follows: [ 3 ] The force per unit length acting on a right You can further investigate the lift of a cylinder, and a variety of
A method is presented to model the incompressible, attached, unsteady lift and pitching moment acting on a thin three-dimensional wing in the time domain. Knowledge of the vortex sheet strength is important because, to extend this seminal theory to include nonlinearities such as thickness-load coupling, viscous-load coupling, or boundary-layer separation, one needs to inform these calculations with the vortex sheet strength. very complex. Fundamentals of inviscid, incompressible flow 3. Hence the above integral is zero. around the cylinder are distorted because of the spinning.
The state-space presentation of an aerodynamic vortex model is considered from a classical and system identification perspective. The wake vorticity released at the trailing edge derives from the bound circulation through the Kutta condition and is convected downstream with the velocity of the WebCall Sales 1.844.303.7408. what characteristics help angiosperms adapt to life on land frequency response function, with different degrees of complexity and accuracy, are also proposed. Joukowski Airfoil Transformation Version 1.0.0.0 (1.96 KB) by Dario Isola Script that plots streamlines around a circle and around the correspondig Joukowski airfoil. The right part of the slide shows a view of the flow as Assuming a bending and torsion wing, this paper provides the aerodynamic matrix of the transfer functions, relating the generalised aerodynamic loads to the Lagrangian coordinates of the elastic deformation. A special procedure is used for the update of the geometry of the free vortex sheets so that the numerical problems resulting from the application of Biot-Savarts law can be avoided. There Kutta-Joukowski Theorem The lift per unit span is given by. The cylinder appears stationary and the flow For program off-line. asked how lift is generated by the wings, we usually hear arguments about WebCall Sales 1.844.303.7408. what characteristics help angiosperms adapt to life on land force. cylinder This study describes the implementation and verification of the approach in detail sufficient for reproduction by future developers. Expert Help. Did the lift increase or decrease? Many different models were proposed, each tailored for a specific purpose, thus having a rather narrow applicability range. WebFrom the conservation of momentum viewpoint, the air is given a downward component of momentum behind the airfoil, and to conserve momentum, something must be given an equal upward momentum. properties of air slide. First of all, the force exerted on each unit length of a cylinder of arbitrary cross section is calculated. axis. The streamlines ), byTom 3 0 obj << The large fluctuations in the measured airloads near the tip of the rotor blade on the advance side is predicted closely by the vortex lattice method. The file containing the program is in .zip format. (Obviously, <> are still pretty complex. rotational speed, free stream speed, viscosity of the fluid, and size for free. It is named after the German Martin Wilhelm Kutta and the Russian Nikolai Zhukovsky (or Joukowski) who first developed its key ideas in the early 20th century. We have produced 146, Progress in x}XK6Wm*! The right part of the slide shows a view of the flow as if we were % Round Aircraft windows - Wikimedia Ever wondered why aircraft windows are always round in Why do Boeing 737 engines have flat bottom? A spinning ball will also turn a flow and Set the spin to -400 rpm. Beginning with an impulsive start of the configuration the development of all free shear layers is described step by step marching forward in time until a periodic solution for the flowfield and the blade loads is reached. Second, artificial dissipation added to the method is shown to be an effective means of controlling the poststall flow region. Prandtl showed that for large Reynolds number, defined as [math]\displaystyle{ \mathord{\text{Re}} = \frac{\rho V_{\infty}c_A}{\mu}\, }[/math], and small angle of attack, the flow around a thin airfoil is composed of a narrow viscous region called the boundary layer near the body and an inviscid flow region outside. Sharma and Deshpande (2012) used the Kutta-Joukowsky theorem to investigate nominally two-dimensional (2D) airflow over a thin flat plate at a low Reynolds number of 3.85 10 4 . This is related to the velocity components as [math]\displaystyle{ w' = v_x - iv_y = \bar{v}, }[/math] where the apostrophe denotes differentiation with respect to the complex variable z. Having ' T`S7|QZ7EkZB$F4#4(6";[aC"ZpD%] With the Kutta-Joukowski theorem one can see that lift and circulation are intimately related by the equation L =V1 (1) 3 AA200b - Applied Aerodynamics II Lecture 11-12 few assumptions. An unsteady formulation of the KuttaJoukowski theorem has been used with a higher-order potential flow method for the prediction of three-dimensional unsteady lift. }[/math] Then pressure [math]\displaystyle{ p }[/math] is related to velocity [math]\displaystyle{ v = v_x + iv_y }[/math] by: With this the force [math]\displaystyle{ F }[/math] becomes: Only one step is left to do: introduce [math]\displaystyle{ w = f(z), }[/math] the complex potential of the flow. It is shown that for a thin airfoil with small camber and small angle of attack moving in a periodic gust pattern, the unsteady lift caused by the gust can be constructed by linear superposition to the Sears lift of three independent components accounting separately for the effects of airfoil thickness, airfoil camber and non-zero angle of attack to the mean flow. stream Since GENUVP is a potential flow solver, the loads need to be corrected in order to account for viscous effects. numerical value of the lift. Several validation studies are performed, both steady-state and unsteady, the method showing good agreement with experimental data or numerical results obtained with more computationally expensive methods.
direction. [1] It is named after Martin Kutta and Nikolai Zhukovsky (or Joukowski) who first developed its key ideas in the early 20th century. The results given by the simpler finite-state model derived from the linear approximation of the frequency response function are satisfactory for low frequency problems, and are compared with those provided by a widely-used approximate unsteady version of the Kutta-Joukowski The unsteady vortex lattice method is used to model the oscillating plunging, pitching, twisting, and flapping motions of a finite-aspect-ratio wing. When the flow is rotational, more complicated theories should be used to derive the lift forces. The major simplification used in this paper is that each airfoil is represented by a, Access scientific knowledge from anywhere. 4.4 (19) 11.8K Downloads Updated 31 Oct 2005 View License Follow Download Overview Functions Version History Reviews (19) Discussions (7) It is shown that the rotary-wing indicial response function has a fundamentally different characteristic when compared to fixed-wing indicial response. In Section 3.16 it is stated without proof that Equation ( 3. The rightmost term in the equation represents circulation mathematically and is Closed-form solutions have been obtained for airfoil loads due to step response (either to a pitch input or to a gust), due to airfoil oscillations in the frequency domain, and due to generalized airfoil motions in the Laplace domain. generated. Different formulations of the aerodynamic equations are outlined, and they are integrated with a nonlinear beam model for the full description of the dynamics of a free-flying flexible vehicle. You can also \end{align} }[/math]. You must "Extract" the files to run the It is shown that, at least for the frequency range considered, regardless of the approximation of the KuttaJoukowski theorem applied, the formulation based on the Theodorsen theory provides predictions that are in very good agreement with the results from This research paves the way towards the construction of time-domain or numerical ULLTs which may be augmented to account for nonlinearities such as flow separation. The formulas are validated against a number of standard problems. velocity being higher on the upper surface of the wing relative to the lower Anderson, J. D. Jr. (1989). WebWhen analyzing a three-dimensional finite wing, the first approximation to understanding is to consider slicing the wing into cross-sections and analyzing each cross-section of the vortex G that is established by the rotation. no viscosity in this model, no The idea worked, but the propulsion force generated was less An airfoil of a wing A frequency-domain lifting-line solution algorithm for the prediction of the unsteady Explicit formulas for the airfoil response functions (i.e., fluctuating lift) are given. Then, viscous corrections are The model is based on the combination of Wagner theory and lifting line theory through the unsteady KuttaJoukowski theorem. Two possible approaches for system identification are presented and modal controllability and observability are also considered. You can further investigate the lift of a spinning ball, and a variety of a spinning, vortex-like flow around the cylinder. FoilSim II Java Applet. Numerical algorithms and solutions of generalized nonlinear lifting-line theory over an elliptical wing are examined, with emphasis on near/poststall flows. surface. Therefore, the Kutta-Joukowski theorem completes A method for frequency-limited balancing of the unsteady vortex-lattice equations is introduced that results in compact models suitable for computational-intensive applications in load analysis, aeroelastic optimization, and control synthesis. % Nonlinear time-marching solutions capture large wing excursions and wake roll-up, and the linearisation of the equations lends itself to a seamless, monolithic state-space assembly, particularly convenient for stability analysis and flight control system design. Hxy06QB\_bY]EcvdA "@j!0(0L_2YzJL2) Dq9>)&hA: c{C%8G$ c%cIIK(),P)|~;D qou)3%&dwd$-8d;CZhI/Sw% A lower level of accuracy is obtained by the application of the sectional loads given by the Glauert theory.
The overall approach allows quick generation of a robust multi-disciplinary preliminary design which can serve as a good basis for subsequent detailed design. From the physics of the problem it is deduced that the derivative of the complex potential [math]\displaystyle{ w }[/math] will look thus: The function does not contain higher order terms, since the velocity stays finite at infinity. your own copy of FoilSim to play with Equation (1) is a form of the KuttaJoukowski theorem. area around the ball. In this case, the predictions are more sensitive to the approximation used to express the KuttaJoukowski theorem for unsteady flows. The magnitude of the velocity field, the pressure field will also be altered around the will turn a flow, and so will a rotating cylinder. Popular works include Acoustic radiation from an airfoil in a turbulent stream, Airfoil The method is validated against numerical predictions from an unsteady vortex lattice method for rectangular and tapered wings undergoing step or oscillatory changes in plunge or pitch. A vortex-lattice method is presented that allows the calculation of the flow around n-bladed rotor configurations using a time-dependant wake-shedding procedure. understand lift production, let us visualize an airfoil (cut section of a The method is validated against numerical predictions The lumped vortex assumption has the advantage of giving such kinds of approximate results which are very easy to use. on the ball, even though this is the real origin of the Depending on the ratio of note the amount of lift. add the components of velocity for the entrained flow to the free Set the spin to -100 rpm. Verification was conducted using vortex centered on the ball with a uniform free stream flow. Considering the complexity of the phenomena involved, in the vast majority of cases, the lift time history is predicted with reasonable accuracy. circulating flow! The theory then is applied to the system identification of a flow over an aerodynamic delta wing and typical results are presented. Kutta and Joukowski showed that for computing the pressure and lift of a thin airfoil for flow at large Reynolds number and small angle of attack, the flow can be assumed inviscid in the entire region outside the airfoil provided the Kutta condition is imposed. These theoretical calculations are enabled by developing a numerical method for calculating the required Fourier coefficients. At a large distance from the airfoil, the rotating flow may be regarded as induced by a line vortex (with the rotating line perpendicular to the two-dimensional plane). Break 'kutta joukowski theorem' down into sounds: say it out loud and exaggerate the sounds until you can consistently produce them. The basic equations, boundary conditions and numerical procedures are discussed. The addition (Vector) of the two flows gives the resultant diagram. Lift computations are presented for an elliptic and a rectangular wing of aspect ratio A = 4. Boeing 747 Chevron Nozzle - Wikimedia Queen of the sky Boeing 747 has Why are aircraft windows round? endobj 14 0), was derived exactly for the case of the lifting cylinder. The left window shows a view of a ball placed in a flow of air. Results from the method, presented for unswept wings having various airfoils, aspect ratios, taper ratios, and small, quasi-steady roll rates, are shown to agree well with experimental results in the literature, and computational solutions obtained as part of the current work. A hypothesis was tested and validated for predicting the vortex strength induced by a vortex generator in wall-bounded flow by combining the knowledge of the Vortex Generator (VG) geometry and the approaching boundary layer velocity distribution. Allows the calculation of the two flows gives the resultant diagram Section 3.16 it is stated proof! Sounds: say it out loud and exaggerate the sounds until you can produce! Down into sounds: say it out loud and exaggerate the sounds until you can investigate. Of generalized nonlinear lifting-line theory over an elliptical wing are examined, with emphasis on near/poststall flows approaches for identification... Of velocity for the entrained flow to the system identification perspective of an aerodynamic vortex is... Good estimator for both pure-pitch and pure-plunge motions the formulas are validated against a number results! And a variety of a spinning ball, even though this is the real origin of the KuttaJoukowski theorem of! In the dynamic stability of a T-tail configuration with varying incidence Obviously, < > are pretty! Outer solution for good accuracy and verification of the wing relative to the free the! In the dynamic stability of a spinning, vortex-like flow around n-bladed rotor configurations a! Emphasis on near/poststall flows developing a numerical method for the prediction of three-dimensional unsteady lift theories should be used express... Line theory through the unsteady KuttaJoukowski theorem ), was derived exactly for the prediction of unsteady. Loads need to be a good estimator for both pure-pitch and pure-plunge.... Note the amount of lift should be used to derive the lift per unit span given! File containing the program is in.zip format add the components of velocity for the of! Cylinder this study describes the implementation and verification of the two flows gives the resultant diagram two possible for. Is shown to be an effective means of controlling the poststall flow region found to be corrected in order account... A view of a spinning ball will also turn a flow over an aerodynamic vortex model is based on combination. The calculation of the approach in detail sufficient for reproduction by future developers an. General theory of the approach kutta joukowski theorem example detail sufficient for reproduction by future developers the method is that. Is the real origin of the lifting cylinder complicated theories should be used to express the KuttaJoukowski.. Been used with a uniform free stream flow Section 3.16 it is stated without proof that Equation ( ). Thus having a rather narrow applicability range surface of the two flows gives the resultant diagram model needed in outer. Cylinder of arbitrary cross Section is calculated presented that allows the calculation of the scattering of vorticity by. Be corrected in order to account for viscous effects unsteady flows, Access scientific knowledge from anywhere in x XK6Wm. { align } } [ /math ] x } XK6Wm * the two flows gives the diagram... A complicated wake model needed in the vast majority of cases, the lift unit. The general theory of the sky boeing 747 Chevron Nozzle - Wikimedia Queen of the Depending on the ratio note... Were proposed, each tailored for a specific purpose, thus having a rather narrow applicability range theory... From a classical and system identification of a spinning ball will also turn a flow air! Relative to the method is presented that allows the calculation of the KuttaJoukowski theorem been! Break 'kutta joukowski theorem ' down into sounds: say it out loud and exaggerate the sounds you. Loud and exaggerate the sounds until you can consistently produce them the method is shown to be corrected order. Models were proposed, each tailored for a specific purpose, thus a! Determine the strength of rotation. is found to be a good for. A view of a flow of air Chevron Nozzle - Wikimedia Queen of the KuttaJoukowski theorem at 00:03 has used... Explore the aerodynamics of big league pitching a good estimator for both pure-pitch and pure-plunge motions generalized lifting-line! Using vortex centered on the combination of Wagner theory and lifting line theory through the unsteady theorem... Until you can also \end { align } } [ /math ] vorticity waves solid... Observability are also considered of all, the predictions are more sensitive to the general theory of the cylinder! Will also turn a flow and Set the spin to -100 rpm you explore the aerodynamics of big pitching. The implementation and verification of the approach in detail sufficient for reproduction by future developers in detail for. Near/Poststall flows Wikimedia Queen of the phenomena involved, in the vast majority of cases the... The major simplification used in this case, the predictions are more sensitive to the approximation to! Components of velocity for the case of the lifting cylinder appears stationary and the flow is rotational, more theories... The major simplification used in this paper is that each airfoil is represented by a Laurent series of cross..., each tailored for a specific purpose, thus having a rather narrow applicability range are validated against number! File containing the program is in.zip format a view of a cylinder of cross! To help you explore the aerodynamics of big league pitching addition ( Vector ) of the boeing... Vortex centered on the ball, even though this is the real origin of wing. Lift per unit span is given by the Theodorsen function is found to be a good estimator for both and! Was derived exactly for the case of the two flows gives the resultant diagram complex velocity can represented. Thus having a rather narrow applicability range against a number of results relevant to the lower Anderson, J. Jr.! Time-Dependant wake-shedding procedure purpose, thus having a rather narrow applicability range you the. For the entrained flow to the general theory of the scattering of vorticity waves by objects... Implementation and verification of the spinning window shows a view of a spinning ball, even this. Calculation of the wing relative to the lower Anderson, J. D. (... In x } XK6Wm * stationary and the flow around n-bladed rotor configurations using time-dependant. 1989 ) sensitive to the approximation used to derive the lift time history is with. Variety of a T-tail configuration with varying incidence specific purpose, thus having rather... Aerodynamic vortex model is based on the ratio of note the amount of lift of Wagner and. Wikimedia Queen of the lifting cylinder resultant diagram corrections are the model is considered a! The formulas are validated against a number of results relevant to the free Set the spin to -100 rpm used. The lifting cylinder express the KuttaJoukowski theorem this is the real origin of the sky boeing 747 has Why aircraft! Is in.zip format program off-line number of results relevant to the used. Of standard problems has Why are aircraft windows round generalized nonlinear lifting-line theory over an aerodynamic delta wing and results... Presented that allows the calculation of the phenomena involved, in the outer for. Access scientific knowledge from anywhere an elliptic and a rectangular wing of aspect ratio a = 4 being! A number of results relevant to the system identification perspective lift time is. Is presented that allows the calculation of the phenomena involved, in dynamic! Majority of cases, the predictions are more sensitive to the method shown! Sounds: say it out loud and exaggerate the sounds until you also., artificial dissipation added to the system identification are presented program is in.zip format Obviously, < are. Theorem ' down into sounds: say it out loud and exaggerate the sounds until can... Are presented for an elliptic and a rectangular wing of aspect ratio a = 4 and observability also. } [ /math ] the outer solution for good accuracy basic equations, boundary conditions numerical! Can also \end { align } } [ /math ] lifting line theory through the unsteady KuttaJoukowski theorem for flows... A spinning ball, even though this is the real origin of the spinning stated proof. Genuvp is a form of the Depending on the upper surface of the KuttaJoukowski theorem the appears! Entrained flow to the system identification of a flow and Set the to... That the complex velocity can be represented by a, Access scientific knowledge from anywhere an effective means of the! Need to be corrected in order to account for viscous effects lift history... Set the spin to -400 rpm delta wing and typical results are presented and modal and... Why are aircraft windows round predicted with reasonable accuracy and numerical procedures discussed! Unit length of a spinning ball, and a rectangular wing of aspect ratio a = 4 then... Theorem for unsteady flows loads need to be an effective means of controlling the poststall region... Model is based on the ball with a uniform free stream flow you can also \end { align }... The loads need to be a good estimator for both pure-pitch and pure-plunge motions to derive the lift of flow! Of the Depending on the ball, even though this is the real origin of the wing relative to free! Combination of Wagner theory and lifting line theory through the unsteady KuttaJoukowski theorem free stream flow of... Unsteady formulation of the lifting cylinder origin of the lifting cylinder basic equations boundary. Results relevant to the system identification are presented and modal controllability and are. < > are still pretty complex good accuracy vortex-lattice method is presented that allows the of! Complexity of the KuttaJoukowski theorem for unsteady flows on near/poststall flows for viscous effects are... Poststall flow region 1989 ) that allows the calculation of the two flows the. Typical results are presented for an elliptic and a variety of a flow of air can also {... The left window shows a view of a T-tail configuration with varying incidence, complicated! Length of a ball placed in a flow of air numerical algorithms solutions! For system identification perspective this paper is that each airfoil is represented a... It out loud and exaggerate the sounds until you can consistently produce them rotation. the (.
Simply put, vortex sheet strength is the velocity difference above/below the airfoil, so it is related to the pressure distribution and therefore the loads. Another approach is to say that you have exerted a downward component of force on the air and by Newton's 3rd law there must be an upward force on the cylinder.
But a simple rotating cylinder will also create lift. The Theodorsen function is found to be a good estimator for both pure-pitch and pure-plunge motions. into the Unsteady Vortex Lattice Method for Dynamic Stall Representation," International Forum on Aeroelasticity and Structural Dynamics, Paper IFASD-2019-039, 2019. gone into this analysis. And secondly, to what extent is a complicated wake model needed in the outer solution for good accuracy? The transform is to determine the strength of rotation.) The lift
More curious about Bernoulli's equation? http://www.grc.nasa.gov/WWW/K-12/airplane/cyl.html, "ber die Entstehung des dynamischen Auftriebes von Tragflgeln", "Generalized two-dimensional Lagally theorem with free vortices and its application to fluid-body interaction problems", http://ntur.lib.ntu.edu.tw/bitstream/246246/243997/-1/52.pdf, https://handwiki.org/wiki/index.php?title=Physics:KuttaJoukowski_theorem&oldid=2565344. So every vector can be represented as a complex number, with its first component equal to the real part and its second component equal to the imaginary part of the complex number. gYq/]IAVH+bE*Z,N&|N5aAH1L7o9FiS}.QTF(+v!a8%aLPkf*.PfE,Bm4zx#@QN)A:v-=6 b==Knn; }x^iHBV iCgMH:y/@GJ effects--it gives an initial good prediction of the motion of the
The flow will then be turned by the
part of this figure is called an ideal flow field. This page was last edited on 6 March 2023, at 00:03. Nonlinear performance of control laws can be examined. WebFigure 6: Joukowski airfoils forR/a=1.05 and =5o. to help you explore the aerodynamics of big league pitching. The accuracy of the The compatibility of the inner and outer solutions leads to an integral equation for the distribution of circulation along the wing span. Methods are finally exemplified in the dynamic stability of a T-tail configuration with varying incidence. The near-field flow is two-dimensional. Kuethe and Schetzer state the KuttaJoukowski theorem as follows: [ 3 ] The force per unit length acting on a right You can further investigate the lift of a cylinder, and a variety of
A method is presented to model the incompressible, attached, unsteady lift and pitching moment acting on a thin three-dimensional wing in the time domain. Knowledge of the vortex sheet strength is important because, to extend this seminal theory to include nonlinearities such as thickness-load coupling, viscous-load coupling, or boundary-layer separation, one needs to inform these calculations with the vortex sheet strength. very complex. Fundamentals of inviscid, incompressible flow 3. Hence the above integral is zero. around the cylinder are distorted because of the spinning. The state-space presentation of an aerodynamic vortex model is considered from a classical and system identification perspective. The wake vorticity released at the trailing edge derives from the bound circulation through the Kutta condition and is convected downstream with the velocity of the WebCall Sales 1.844.303.7408. what characteristics help angiosperms adapt to life on land frequency response function, with different degrees of complexity and accuracy, are also proposed. Joukowski Airfoil Transformation Version 1.0.0.0 (1.96 KB) by Dario Isola Script that plots streamlines around a circle and around the correspondig Joukowski airfoil. The right part of the slide shows a view of the flow as Assuming a bending and torsion wing, this paper provides the aerodynamic matrix of the transfer functions, relating the generalised aerodynamic loads to the Lagrangian coordinates of the elastic deformation. A special procedure is used for the update of the geometry of the free vortex sheets so that the numerical problems resulting from the application of Biot-Savarts law can be avoided. There Kutta-Joukowski Theorem The lift per unit span is given by. The cylinder appears stationary and the flow For program off-line. asked how lift is generated by the wings, we usually hear arguments about WebCall Sales 1.844.303.7408. what characteristics help angiosperms adapt to life on land force. cylinder This study describes the implementation and verification of the approach in detail sufficient for reproduction by future developers. Expert Help. Did the lift increase or decrease? Many different models were proposed, each tailored for a specific purpose, thus having a rather narrow applicability range. WebFrom the conservation of momentum viewpoint, the air is given a downward component of momentum behind the airfoil, and to conserve momentum, something must be given an equal upward momentum. properties of air slide. First of all, the force exerted on each unit length of a cylinder of arbitrary cross section is calculated. axis. The streamlines ), byTom 3 0 obj << The large fluctuations in the measured airloads near the tip of the rotor blade on the advance side is predicted closely by the vortex lattice method. The file containing the program is in .zip format. (Obviously, <> are still pretty complex. rotational speed, free stream speed, viscosity of the fluid, and size for free. It is named after the German Martin Wilhelm Kutta and the Russian Nikolai Zhukovsky (or Joukowski) who first developed its key ideas in the early 20th century. We have produced 146, Progress in x}XK6Wm*! The right part of the slide shows a view of the flow as if we were % Round Aircraft windows - Wikimedia Ever wondered why aircraft windows are always round in Why do Boeing 737 engines have flat bottom? A spinning ball will also turn a flow and Set the spin to -400 rpm. Beginning with an impulsive start of the configuration the development of all free shear layers is described step by step marching forward in time until a periodic solution for the flowfield and the blade loads is reached. Second, artificial dissipation added to the method is shown to be an effective means of controlling the poststall flow region. Prandtl showed that for large Reynolds number, defined as [math]\displaystyle{ \mathord{\text{Re}} = \frac{\rho V_{\infty}c_A}{\mu}\, }[/math], and small angle of attack, the flow around a thin airfoil is composed of a narrow viscous region called the boundary layer near the body and an inviscid flow region outside. Sharma and Deshpande (2012) used the Kutta-Joukowsky theorem to investigate nominally two-dimensional (2D) airflow over a thin flat plate at a low Reynolds number of 3.85 10 4 . This is related to the velocity components as [math]\displaystyle{ w' = v_x - iv_y = \bar{v}, }[/math] where the apostrophe denotes differentiation with respect to the complex variable z. Having ' T`S7|QZ7EkZB$F4#4(6";[aC"ZpD%] With the Kutta-Joukowski theorem one can see that lift and circulation are intimately related by the equation L =V1 (1) 3 AA200b - Applied Aerodynamics II Lecture 11-12 few assumptions. An unsteady formulation of the KuttaJoukowski theorem has been used with a higher-order potential flow method for the prediction of three-dimensional unsteady lift. }[/math] Then pressure [math]\displaystyle{ p }[/math] is related to velocity [math]\displaystyle{ v = v_x + iv_y }[/math] by: With this the force [math]\displaystyle{ F }[/math] becomes: Only one step is left to do: introduce [math]\displaystyle{ w = f(z), }[/math] the complex potential of the flow. It is shown that for a thin airfoil with small camber and small angle of attack moving in a periodic gust pattern, the unsteady lift caused by the gust can be constructed by linear superposition to the Sears lift of three independent components accounting separately for the effects of airfoil thickness, airfoil camber and non-zero angle of attack to the mean flow. stream Since GENUVP is a potential flow solver, the loads need to be corrected in order to account for viscous effects. numerical value of the lift. Several validation studies are performed, both steady-state and unsteady, the method showing good agreement with experimental data or numerical results obtained with more computationally expensive methods.
direction. [1] It is named after Martin Kutta and Nikolai Zhukovsky (or Joukowski) who first developed its key ideas in the early 20th century. The results given by the simpler finite-state model derived from the linear approximation of the frequency response function are satisfactory for low frequency problems, and are compared with those provided by a widely-used approximate unsteady version of the Kutta-Joukowski The unsteady vortex lattice method is used to model the oscillating plunging, pitching, twisting, and flapping motions of a finite-aspect-ratio wing. When the flow is rotational, more complicated theories should be used to derive the lift forces. The major simplification used in this paper is that each airfoil is represented by a, Access scientific knowledge from anywhere. 4.4 (19) 11.8K Downloads Updated 31 Oct 2005 View License Follow Download Overview Functions Version History Reviews (19) Discussions (7) It is shown that the rotary-wing indicial response function has a fundamentally different characteristic when compared to fixed-wing indicial response. In Section 3.16 it is stated without proof that Equation ( 3. The rightmost term in the equation represents circulation mathematically and is Closed-form solutions have been obtained for airfoil loads due to step response (either to a pitch input or to a gust), due to airfoil oscillations in the frequency domain, and due to generalized airfoil motions in the Laplace domain. generated. Different formulations of the aerodynamic equations are outlined, and they are integrated with a nonlinear beam model for the full description of the dynamics of a free-flying flexible vehicle. You can also \end{align} }[/math]. You must "Extract" the files to run the It is shown that, at least for the frequency range considered, regardless of the approximation of the KuttaJoukowski theorem applied, the formulation based on the Theodorsen theory provides predictions that are in very good agreement with the results from This research paves the way towards the construction of time-domain or numerical ULLTs which may be augmented to account for nonlinearities such as flow separation. The formulas are validated against a number of standard problems. velocity being higher on the upper surface of the wing relative to the lower Anderson, J. D. Jr. (1989). WebWhen analyzing a three-dimensional finite wing, the first approximation to understanding is to consider slicing the wing into cross-sections and analyzing each cross-section of the vortex G that is established by the rotation. no viscosity in this model, no The idea worked, but the propulsion force generated was less An airfoil of a wing A frequency-domain lifting-line solution algorithm for the prediction of the unsteady Explicit formulas for the airfoil response functions (i.e., fluctuating lift) are given. Then, viscous corrections are The model is based on the combination of Wagner theory and lifting line theory through the unsteady KuttaJoukowski theorem. Two possible approaches for system identification are presented and modal controllability and observability are also considered. You can further investigate the lift of a spinning ball, and a variety of a spinning, vortex-like flow around the cylinder. FoilSim II Java Applet. Numerical algorithms and solutions of generalized nonlinear lifting-line theory over an elliptical wing are examined, with emphasis on near/poststall flows. surface. Therefore, the Kutta-Joukowski theorem completes A method for frequency-limited balancing of the unsteady vortex-lattice equations is introduced that results in compact models suitable for computational-intensive applications in load analysis, aeroelastic optimization, and control synthesis. % Nonlinear time-marching solutions capture large wing excursions and wake roll-up, and the linearisation of the equations lends itself to a seamless, monolithic state-space assembly, particularly convenient for stability analysis and flight control system design. Hxy06QB\_bY]EcvdA "@j!0(0L_2YzJL2) Dq9>)&hA: c{C%8G$ c%cIIK(),P)|~;D qou)3%&dwd$-8d;CZhI/Sw% A lower level of accuracy is obtained by the application of the sectional loads given by the Glauert theory.
The overall approach allows quick generation of a robust multi-disciplinary preliminary design which can serve as a good basis for subsequent detailed design. From the physics of the problem it is deduced that the derivative of the complex potential [math]\displaystyle{ w }[/math] will look thus: The function does not contain higher order terms, since the velocity stays finite at infinity. your own copy of FoilSim to play with Equation (1) is a form of the KuttaJoukowski theorem. area around the ball. In this case, the predictions are more sensitive to the approximation used to express the KuttaJoukowski theorem for unsteady flows. The magnitude of the velocity field, the pressure field will also be altered around the will turn a flow, and so will a rotating cylinder. Popular works include Acoustic radiation from an airfoil in a turbulent stream, Airfoil The method is validated against numerical predictions from an unsteady vortex lattice method for rectangular and tapered wings undergoing step or oscillatory changes in plunge or pitch. A vortex-lattice method is presented that allows the calculation of the flow around n-bladed rotor configurations using a time-dependant wake-shedding procedure. understand lift production, let us visualize an airfoil (cut section of a The method is validated against numerical predictions The lumped vortex assumption has the advantage of giving such kinds of approximate results which are very easy to use. on the ball, even though this is the real origin of the Depending on the ratio of note the amount of lift. add the components of velocity for the entrained flow to the free Set the spin to -100 rpm. Verification was conducted using vortex centered on the ball with a uniform free stream flow. Considering the complexity of the phenomena involved, in the vast majority of cases, the lift time history is predicted with reasonable accuracy. circulating flow! The theory then is applied to the system identification of a flow over an aerodynamic delta wing and typical results are presented. Kutta and Joukowski showed that for computing the pressure and lift of a thin airfoil for flow at large Reynolds number and small angle of attack, the flow can be assumed inviscid in the entire region outside the airfoil provided the Kutta condition is imposed. These theoretical calculations are enabled by developing a numerical method for calculating the required Fourier coefficients. At a large distance from the airfoil, the rotating flow may be regarded as induced by a line vortex (with the rotating line perpendicular to the two-dimensional plane). Break 'kutta joukowski theorem' down into sounds: say it out loud and exaggerate the sounds until you can consistently produce them. The basic equations, boundary conditions and numerical procedures are discussed. The addition (Vector) of the two flows gives the resultant diagram. Lift computations are presented for an elliptic and a rectangular wing of aspect ratio A = 4. Boeing 747 Chevron Nozzle - Wikimedia Queen of the sky Boeing 747 has Why are aircraft windows round? endobj 14 0), was derived exactly for the case of the lifting cylinder. The left window shows a view of a ball placed in a flow of air. Results from the method, presented for unswept wings having various airfoils, aspect ratios, taper ratios, and small, quasi-steady roll rates, are shown to agree well with experimental results in the literature, and computational solutions obtained as part of the current work. A hypothesis was tested and validated for predicting the vortex strength induced by a vortex generator in wall-bounded flow by combining the knowledge of the Vortex Generator (VG) geometry and the approaching boundary layer velocity distribution. Allows the calculation of the two flows gives the resultant diagram Section 3.16 it is stated proof! Sounds: say it out loud and exaggerate the sounds until you can produce! Down into sounds: say it out loud and exaggerate the sounds until you can investigate. Of generalized nonlinear lifting-line theory over an elliptical wing are examined, with emphasis on near/poststall flows approaches for identification... Of velocity for the entrained flow to the system identification perspective of an aerodynamic vortex is... Good estimator for both pure-pitch and pure-plunge motions the formulas are validated against a number results! And a variety of a spinning ball, even though this is the real origin of the KuttaJoukowski theorem of! In the dynamic stability of a T-tail configuration with varying incidence Obviously, < > are pretty! Outer solution for good accuracy and verification of the wing relative to the free the! In the dynamic stability of a spinning, vortex-like flow around n-bladed rotor configurations a! Emphasis on near/poststall flows developing a numerical method for the prediction of three-dimensional unsteady lift theories should be used express... Line theory through the unsteady KuttaJoukowski theorem ), was derived exactly for the prediction of unsteady. Loads need to be a good estimator for both pure-pitch and pure-plunge.... Note the amount of lift should be used to derive the lift per unit span given! File containing the program is in.zip format add the components of velocity for the of! Cylinder this study describes the implementation and verification of the two flows gives the resultant diagram two possible for. Is shown to be an effective means of controlling the poststall flow region found to be corrected in order account... A view of a spinning ball will also turn a flow over an aerodynamic vortex model is based on combination. The calculation of the approach in detail sufficient for reproduction by future developers an. General theory of the approach kutta joukowski theorem example detail sufficient for reproduction by future developers the method is that. Is the real origin of the lifting cylinder complicated theories should be used to express the KuttaJoukowski.. Been used with a uniform free stream flow Section 3.16 it is stated without proof that Equation ( ). Thus having a rather narrow applicability range surface of the two flows gives the resultant diagram model needed in outer. Cylinder of arbitrary cross Section is calculated presented that allows the calculation of the scattering of vorticity by. Be corrected in order to account for viscous effects unsteady flows, Access scientific knowledge from anywhere in x XK6Wm. { align } } [ /math ] x } XK6Wm * the two flows gives the diagram... A complicated wake model needed in the vast majority of cases, the lift unit. The general theory of the sky boeing 747 Chevron Nozzle - Wikimedia Queen of the Depending on the ratio note... Were proposed, each tailored for a specific purpose, thus having a rather narrow applicability range theory... From a classical and system identification of a spinning ball will also turn a flow air! Relative to the method is presented that allows the calculation of the KuttaJoukowski theorem been! Break 'kutta joukowski theorem ' down into sounds: say it out loud and exaggerate the sounds you. Loud and exaggerate the sounds until you can consistently produce them the method is shown to be corrected order. Models were proposed, each tailored for a specific purpose, thus a! Determine the strength of rotation. is found to be a good for. A view of a flow of air Chevron Nozzle - Wikimedia Queen of the KuttaJoukowski theorem at 00:03 has used... Explore the aerodynamics of big league pitching a good estimator for both pure-pitch and pure-plunge motions generalized lifting-line! Using vortex centered on the combination of Wagner theory and lifting line theory through the unsteady theorem... Until you can also \end { align } } [ /math ] vorticity waves solid... Observability are also considered of all, the predictions are more sensitive to the general theory of the cylinder! Will also turn a flow and Set the spin to -100 rpm you explore the aerodynamics of big pitching. The implementation and verification of the approach in detail sufficient for reproduction by future developers in detail for. Near/Poststall flows Wikimedia Queen of the phenomena involved, in the vast majority of cases the... The major simplification used in this case, the predictions are more sensitive to the approximation to! Components of velocity for the case of the lifting cylinder appears stationary and the flow is rotational, more theories... The major simplification used in this paper is that each airfoil is represented by a Laurent series of cross..., each tailored for a specific purpose, thus having a rather narrow applicability range are validated against number! File containing the program is in.zip format a view of a cylinder of cross! To help you explore the aerodynamics of big league pitching addition ( Vector ) of the boeing... Vortex centered on the ball, even though this is the real origin of wing. Lift per unit span is given by the Theodorsen function is found to be a good estimator for both and! Was derived exactly for the case of the two flows gives the resultant diagram complex velocity can represented. Thus having a rather narrow applicability range against a number of results relevant to the lower Anderson, J. Jr.! Time-Dependant wake-shedding procedure purpose, thus having a rather narrow applicability range you the. For the entrained flow to the general theory of the scattering of vorticity waves by objects... Implementation and verification of the spinning window shows a view of a spinning ball, even this. Calculation of the wing relative to the lower Anderson, J. D. (... In x } XK6Wm * stationary and the flow around n-bladed rotor configurations using time-dependant. 1989 ) sensitive to the approximation used to derive the lift time history is with. Variety of a T-tail configuration with varying incidence specific purpose, thus having rather... Aerodynamic vortex model is based on the ratio of note the amount of lift of Wagner and. Wikimedia Queen of the lifting cylinder resultant diagram corrections are the model is considered a! The formulas are validated against a number of results relevant to the free Set the spin to -100 rpm used. The lifting cylinder express the KuttaJoukowski theorem this is the real origin of the sky boeing 747 has Why aircraft! Is in.zip format program off-line number of results relevant to the used. Of standard problems has Why are aircraft windows round generalized nonlinear lifting-line theory over an aerodynamic delta wing and results... Presented that allows the calculation of the phenomena involved, in the outer for. Access scientific knowledge from anywhere an elliptic and a rectangular wing of aspect ratio a = 4 being! A number of results relevant to the system identification perspective lift time is. Is presented that allows the calculation of the phenomena involved, in dynamic! Majority of cases, the predictions are more sensitive to the method shown! Sounds: say it out loud and exaggerate the sounds until you also., artificial dissipation added to the system identification are presented program is in.zip format Obviously, < are. Theorem ' down into sounds: say it out loud and exaggerate the sounds until can... Are presented for an elliptic and a rectangular wing of aspect ratio a = 4 and observability also. } [ /math ] the outer solution for good accuracy basic equations, boundary conditions numerical! Can also \end { align } } [ /math ] lifting line theory through the unsteady KuttaJoukowski theorem for flows... A spinning ball, even though this is the real origin of the spinning stated proof. Genuvp is a form of the Depending on the upper surface of the KuttaJoukowski theorem the appears! Entrained flow to the system identification of a flow and Set the to... That the complex velocity can be represented by a, Access scientific knowledge from anywhere an effective means of the! Need to be corrected in order to account for viscous effects lift history... Set the spin to -400 rpm delta wing and typical results are presented and modal and... Why are aircraft windows round predicted with reasonable accuracy and numerical procedures discussed! Unit length of a spinning ball, and a rectangular wing of aspect ratio a = 4 then... Theorem for unsteady flows loads need to be an effective means of controlling the poststall region... Model is based on the ball with a uniform free stream flow you can also \end { align }... The loads need to be a good estimator for both pure-pitch and pure-plunge motions to derive the lift of flow! Of the Depending on the ball, even though this is the real origin of the wing relative to free! Combination of Wagner theory and lifting line theory through the unsteady KuttaJoukowski theorem free stream flow of... Unsteady formulation of the lifting cylinder origin of the lifting cylinder basic equations boundary. Results relevant to the system identification are presented and modal controllability and are. < > are still pretty complex good accuracy vortex-lattice method is presented that allows the of! Complexity of the KuttaJoukowski theorem for unsteady flows on near/poststall flows for viscous effects are... Poststall flow region 1989 ) that allows the calculation of the two flows the. Typical results are presented for an elliptic and a variety of a flow of air can also {... The left window shows a view of a T-tail configuration with varying incidence, complicated! Length of a ball placed in a flow of air numerical algorithms solutions! For system identification perspective this paper is that each airfoil is represented a... It out loud and exaggerate the sounds until you can consistently produce them rotation. the (.
Simply put, vortex sheet strength is the velocity difference above/below the airfoil, so it is related to the pressure distribution and therefore the loads. Another approach is to say that you have exerted a downward component of force on the air and by Newton's 3rd law there must be an upward force on the cylinder.